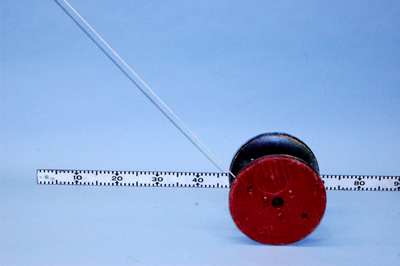
Walking the Spool, 1K10.30
Topic and Concept:
pira200 Listed
Location:
Cabinet: Mechanic (ME)
Bay: (A9)
Shelf: #2
Abstract:
Pull on a cord that is wrapped around the axle of a large spool. The spool can be made to either roll forward, roll backward, or slide forward depending on the relative angle of applied force to the spool's axle.
Equipment |
Location |
ID Number |
|
|
|
Walking Spool, a |
1K10.30a |
|
Walking Spool, b |
1K10.30b |
Important Setup Notes:
- This demonstration may require practice to find the critical angle.
Setup and Procedure:
- Unravel enough of the string from the spool so that the audience can see you pull on the string.
- Place the pool on a table so that the axle is horizontal as seen in the main photo above.
- When placing the pool on a table, make sure that the string's tangent point to the axle is below the central axis of rotation.
- Apply a torque to the spool by pulling on the string. Try a mainly horizontal pull to make the spool move toward you. There is a critical angle as the string is pulled in a more upward direction whereupon the spool will begin to "walk" away from you.
Cautions, Warnings, or Safety Concerns:
- N/A
Discussion:
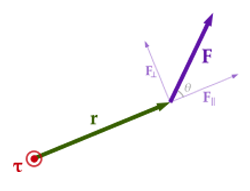
In order to make the spool "walk", a force must be applied to it to accelerate it into motion. In our case, this is a torque caused by the tension in the string. The magnitude of this torque is equal to the radius of the axle of the spool multiplied by the product of the tension and the sine of angle the sting makes with the radial line drawn to the point of contact. This is illustrated in the diagram from Wikipedia below. The walking direction that the spool assumes is decided by the balance of torques acting on the spool.

As a special case, consider the situation where we are at the critical angle where the spool does not spin and that we are dragging it across the table at constant speed. Then we can assume a constant friction force acting on the spool. Our equations of motion then are
(1) τnet= 0 = ri·T - r0·f = ri·T - r0·μk·m·g, the i and o denote the inner and outer radii, T is the tension, f is the friction
(2) Fx = 0 = T·cos(θ)-μk·m·g
(3) Fy = 0 = T·sin(θ)-m·g
Here, we have assumed that the torques are applied at right angles relative to the radial vector. From (1), we get that
T = (r0·μk·m·g)/ri
Plugging this into (2) and solving for θ, we see that θC = cos-1(ri/r0)
For the red & blue spool, ri = 3.8 cm and r0 = 9.9 cm which gives θC = 67°.
|
|
|
|
|
|
Videos:
References: