|
Size: 1755
Comment:
|
← Revision 7 as of 2013-07-12 18:17:35 ⇥
Size: 2232
Comment: converted to 1.6 markup
|
| Deletions are marked like this. | Additions are marked like this. |
| Line 2: | Line 2: |
| ||<:30%>[:PiraScheme#Mechanics: Table of Mechanics Demonstration]||<:30%>[:MEEquipmentList: List of Mechanics Equipment & Supplies]||<:30%>[:Demonstrations:Lecture Demonstrations]|| | ||<:30%>[[PiraScheme#WavesSound| Table of Waves and Sound Demonstration]]||<:30%>[[MEEquipmentList| List of Mechanics Equipment & Supplies]]||<:30%>[[Demonstrations|Lecture Demonstrations]]|| |
| Line 7: | Line 7: |
| Oscillations, [:Oscillations#Nonlinear: 3A95. Non-Linear Systems] | Oscillations, [[Oscillations#Nonlinear| 3A95. Non-Linear Systems]] |
| Line 10: | Line 10: |
| * '''Cabinet:''' [:MechanicsCabinet:Mechanic (ME)] * '''Bay:''' [:MechanicsCabinetBayA1:(A1)] * '''Shelf:''' #1,2,3.. |
* '''Cabinet:''' [[MechanicsCabinet|Mechanic (ME)]] * '''Bay:''' (A13) * '''Shelf:''' #13 |
| Line 14: | Line 14: |
| attachment:AnharmonicPingPongBall05-400.jpg | {{attachment:AnharmonicPingPongBall05-400.jpg}} |
| Line 22: | Line 22: |
| ||apparatus||[:MechanicsCabinetBayB1: ME, Bay B1, Shelf #2]|| || ||all other parts||[:MechanicsCabinetBayB1: ME, Bay B1, Shelf #2]|| || ||...||[:MechanicsCabinetBayA5: ME, Bay A5, Shelf #2]|| || |
||Ping Pong Balls||ME, Bay A13, Shelf #3|| || |
| Line 33: | Line 30: |
| 1. List steps for setup then procedure. 1. ... |
1. Drop the ping pong ball from some height onto a table, and observe the decay in round-trip time. |
| Line 42: | Line 38: |
| Discuss the physics behind the demonstration, explaining some of the various steps of the demonstration when appropriate. | Let's define an oscillation of this system as the drop from maximum height, a bounce, and a return of the ping pong ball to a new maximum height. The acceleration of the ping pong ball is nearly always constant with the exception of the time spent in contact with the tabletop. We notice that, with each oscillation, the maximum height the ball reaches decreases monotonically with time. This is because energy is dissipated with each collision of the ball with the table. Thus, the oscillatory nature of this system cannot be described with a single frequency of oscillation. This is what makes this system anharmonic. |
| Line 44: | Line 40: |
| ||attachment:AnharmonicPingPongBall01-250.jpg||attachment:AnharmonicPingPongBall02-250.jpg||attachment:AnharmonicPingPongBall03-250.jpg||attachment:AnharmonicPingPongBall04-250.jpg|| | ||{{attachment:AnharmonicPingPongBall01-250.jpg}}||{{attachment:AnharmonicPingPongBall02-250.jpg}}||{{attachment:AnharmonicPingPongBall03-250.jpg}}||{{attachment:AnharmonicPingPongBall04-250.jpg}}|| |
| Line 47: | Line 43: |
| * [https://www.youtube.com/user/LectureDemostrations/videos?view=1 Lecture Demonstration's Youtube Channel] | * [[https://www.youtube.com/user/LectureDemostrations/videos?view=1|Lecture Demonstration's Youtube Channel]] |
| Line 51: | Line 47: |
| * [https://en.wikipedia.org/wiki/Anharmonicity Wikipedia - Anharmonicity] | * [[https://en.wikipedia.org/wiki/Anharmonicity|Wikipedia - Anharmonicity]] * [[https://en.wikipedia.org/wiki/Oscillation|Wikipedia - Oscillation]] |
| Line 54: | Line 51: |
| [:Instructional:Home] | [[Instructional|Home]] |
Anharmonic Ping Pong Ball, 3A95.40
Topic and Concept:
Oscillations, 3A95. Non-Linear Systems
Location:
Cabinet: Mechanic (ME)
Bay: (A13)
Shelf: #13
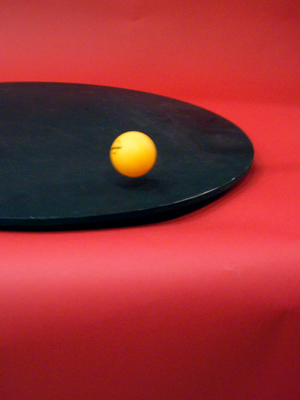
Abstract:
A ping pong ball is dropped and then bounces anharmonically.
Equipment |
Location |
ID Number |
|
|
|
Ping Pong Balls |
ME, Bay A13, Shelf #3 |
|
Important Setup Notes:
- N/A
Setup and Procedure:
- Drop the ping pong ball from some height onto a table, and observe the decay in round-trip time.
Cautions, Warnings, or Safety Concerns:
- N/A
Discussion:
Let's define an oscillation of this system as the drop from maximum height, a bounce, and a return of the ping pong ball to a new maximum height. The acceleration of the ping pong ball is nearly always constant with the exception of the time spent in contact with the tabletop. We notice that, with each oscillation, the maximum height the ball reaches decreases monotonically with time. This is because energy is dissipated with each collision of the ball with the table. Thus, the oscillatory nature of this system cannot be described with a single frequency of oscillation. This is what makes this system anharmonic.
|
|
|
|
Videos:
References:



